Lesson: Chapter - 8

Springs

Questions about springs on subject test Physics are usually simple matters of a mass on a spring oscillating back and forth. However, spring motion is the most interesting of the four topics we will cover here because of its generality. The harmonic motion that springs exhibit applies equally to objects moving in a circular path and to the various wave phenomena that we’ll study later in this book. So before we dig in to the nitty-gritty of your typical subject test Physics spring questions, let’s look at some general features of harmonic motion.

Oscillation and Harmonic Motion

Consider the following physical phenomena:

• When you drop a rock into a still pond, the rock makes a big splash, which causes ripples to spread out to the edges of the pond.
• When you pluck a guitar string, the string vibrates back and forth.
• When you rock a small boat, it wobbles to and fro in the water before coming to rest again.
• When you stretch out a spring and release it, the spring goes back and forth between being compressed and being stretched out.

There are just a few examples of the widespread phenomenon of oscillation. Oscillation is the natural world’s way of returning a system to its equilibrium position, the stable position of the system where the net force acting on it is zero. If you throw a system off-balance, it doesn’t simply return to the way it was; it oscillates back and forth about the equilibrium position.

A system oscillates as a way of giving off energy. A system that is thrown off-kilter has more energy than a system in its equilibrium position. To take the simple example of a spring, a stretched-out spring will start to move as soon as you let go of it: that motion is evidence of kinetic energy that the spring lacks in its equilibrium position. Because of the law of conservation of energy, a stretched-out spring cannot simply return to its equilibrium position; it must release some energy in order to do so. Usually, this energy is released as thermal energy caused by friction, but there are plenty of interesting exceptions. For instance, a plucked guitar string releases sound energy: the music we hear is the result of the string returning to its equilibrium position.

The movement of an oscillating body is called harmonic motion. If you were to graph the position, velocity, or acceleration of an oscillating body against time, the result would be a sinusoidal wave; that is, some variation of a y = a sin bx or a y = a cos bx graph. This generalized form of harmonic motion applies not only to springs and guitar strings, but to anything that moves in a cycle. Imagine placing a pebble on the edge of a turntable, and watching the turntable rotate while looking at it from the side. You will see the pebble moving back and forth in one dimension. The pebble will appear to oscillate just like a spring: it will appear to move fastest at the middle of its trajectory and slow to a halt and reverse direction as it reaches the edge of its trajectory.

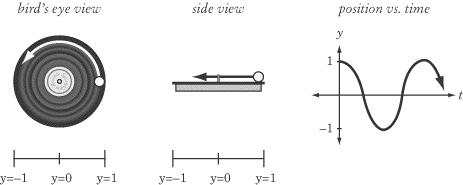
This example serves two purposes. First, it shows you that the oscillation of springs is just one of a wide range of phenomena exhibiting harmonic motion. Anything that moves in a cyclic pattern exhibits harmonic motion. This includes the light and sound waves without which we would have a lot of trouble moving about in the world. Second, we bring it up because subject test Physics has been known to test students on the nature of the horizontal or vertical component of the motion of an object in circular motion. As you can see, circular motion viewed in one dimension is harmonic motion.

Though harmonic motion is one of the most widespread and important of physical phenomena, your understanding of it will not be taxed to any great extent on SAT II Physics. In fact, beyond the motion of springs and pendulums, everything you will need to know will be covered in this book in the chapter on Waves. The above discussion is mostly meant to fit your understanding of the oscillation of springs into a wider context.

The Oscillation of a Spring

Now let’s focus on the harmonic motion exhibited by a spring. To start with, we’ll imagine a mass, m, placed on a frictionless surface, and attached to a wall by a spring. In its equilibrium position, where no forces act upon it, the mass is at rest. Let’s label this equilibrium position x = 0. Intuitively, you know that if you compress or stretch out the spring it will begin to oscillate.

Suppose you push the mass toward the wall, compressing the spring, until the mass is in position x = x_min .

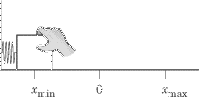
When you release the mass, the spring will exert a force, pushing the mass back until it reaches position , which is called the amplitude of the spring’s motion, or the maximum displacement of the oscillator. Note that . x_min = -x_max

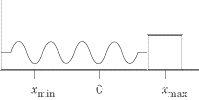
By that point, the spring will be stretched out, and will be exerting a force to pull the mass back in toward the wall. Because we are dealing with an idealized frictionless surface, the mass will not be slowed by the force of friction, and will oscillate back and forth repeatedly between x_min and x_max.

Hooke’s Law

This is all well and good, but we can’t get very far in sorting out the amplitude, the velocity, the energy, or anything else about the mass’s motion if we don’t understand the manner in which the spring exerts a force on the mass attached to it. The force, F, that the spring exerts on the mass is defined by Hooke’s Law:

F = -kz

where x is the spring’s displacement from its equilibrium position and k is a constant of proportionality called the spring constant. The spring constant is a measure of “springiness”: a greater value for k signifies a “tighter” spring, one that is more resistant to being stretched.

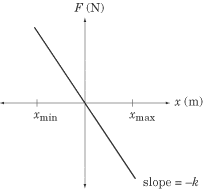
Hooke’s Law tells us that the further the spring is displaced from its equilibrium position (x) the greater the force the spring will exert in the direction of its equilibrium position (F). We call F a restoring force: it is always directed toward equilibrium. Because F and x are directly proportional, a graph of F vs. x is a line with slope –k.

Simple Harmonic Oscillation

A mass oscillating on a spring is one example of a simple harmonic oscillator. Specifically, a simple harmonic oscillator is any object that moves about a stable equilibrium point and experiences a restoring force proportional to the oscillator’s displacement.

For an oscillating spring, the restoring force, and consequently the acceleration, are greatest and positive at x_min. These quantities decrease as x approaches the equilibrium position and are zero at x = 0. The restoring force and acceleration—which are now negative—increase in magnitude as x approaches x_max and are maximally negative at x_max.

Important Properties of a Mass on a Spring

There are a number of important properties related to the motion of a mass on a spring, all of which are fair game for subject test Physics. Remember, though: the test makers have no interest in testing your ability to recall complex formulas and perform difficult mathematical operations. You may be called upon to know the simpler of these formulas, but not the complex ones. As we mentioned at the end of the section on pulleys, it’s less important that you memorize the formulas and more important that you understand what they mean. If you understand the principle, there probably won’t be any questions that will stump you.

Period of Oscillation

The period of oscillation, T, of a spring is the amount of time it takes for a spring to complete a round-trip or cycle. Mathematically, the period of oscillation of a simple harmonic oscillator described by Hooke’s Law is:

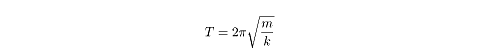
This equation tells us that as the mass of the block, m, increases and the spring constant, k, decreases, the period increases. In other words, a heavy mass attached to an easily stretched spring will oscillate back and forth very slowly, while a light mass attached to a resistant spring will oscillate back and forth very quickly.

Frequency

The frequency of the spring’s motion tells us how quickly the object is oscillating, or how many cycles it completes in a given timeframe. Frequency is inversely proportional to period:

f = 1/T

Frequency is given in units of cycles per second, or hertz (Hz).

Potential Energy

The potential energy of a spring (U_s) is sometimes called elastic energy, because it results from the spring being stretched or compressed. Mathematically, U_s is defined by:

U = 1/2 kx²

The potential energy of a spring is greatest when the coil is maximally compressed or stretched, and is zero at the equilibrium position.

Kinetic Energy

subject test Physics will not test you on the motion of springs involving friction, so for the purposes of the test, the mechanical energy of a spring is a conserved quantity. As we recall, mechanical energy is the sum of the kinetic energy and potential energy.

At the points of maximum compression and extension, the velocity, and hence the kinetic energy, is zero and the mechanical energy is equal to the potential energy, U_s= ¹/₂1/2 kx².

At the equilibrium position, the potential energy is zero, and the velocity and kinetic energy are maximized. The kinetic energy at the equilibrium position is equal to the mechanical energy:

KE_max = mv² = 1/2 kx²_max

From this equation, we can derive the maximum velocity:

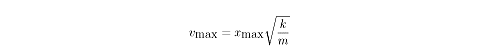
You won’t need to know this equation, but it might be valuable to note that the velocity increases with a large displacement, a resistant spring, and a small mass.

Summary

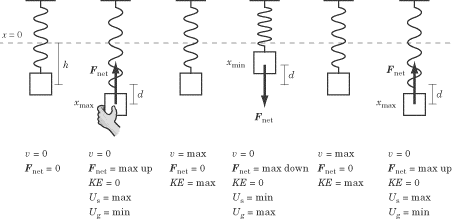
It is highly unlikely that the formulas discussed above will appear on subject test Physics. More likely, you will be asked conceptual questions such as: at what point in a spring’s oscillation is the kinetic or potential energy maximized or minimized, for instance. The figure below summarizes and clarifies some qualitative aspects of simple harmonic oscillation. Your qualitative understanding of the relationship between force, velocity, and kinetic and potential energy in a spring system is far more likely to be tested than your knowledge of the formulas discussed above.

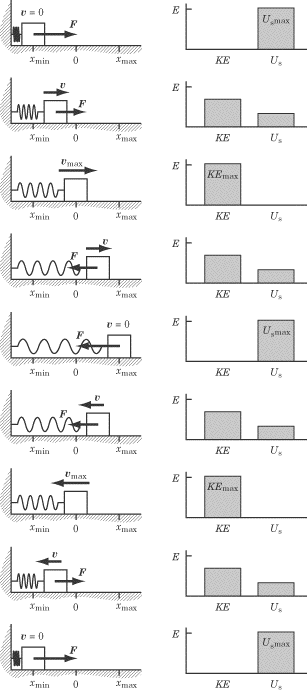
In this figure, v represents velocity, F represents force, KE represents kinetic energy, U_s and represents potential energy.

Vertical Oscillation of Springs

Now let’s consider a mass attached to a spring that is suspended from the ceiling. Questions of this sort have a nasty habit of coming up on subject test Physics. The oscillation of the spring when compressed or extended won’t be any different, but we now have to take gravity into account.

Equilibrium Position

Because the mass will exert a gravitational force to stretch the spring downward a bit, the equilibrium position will no longer be atx = 0, but at x = –h, where h is the vertical displacement of the spring due to the gravitational pull exerted on the mass. The equilibrium position is the point where the net force acting on the mass is zero; in other words, the point where the upward restoring force of the spring is equal to the downward gravitational force of the mass.

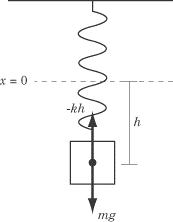
Combining the restoring force, F = –kh, and the gravitational force, F = mg, we can solve for h:

-kh = mg
-h = mg/k

Since m is in the numerator and k in the denominator of the fraction, the mass displaces itself more if it has a large weight and is suspended from a lax spring, as intuition suggests.

A Vertical Spring in Motion

If the spring is then stretched a distance d, where d < h, it will oscillate between X_max = -b - d and X_min = -b + d.

Throughout the motion of the mass, the force of gravity is constant and downward. The restoring force of the spring is always upward, because even at X_min the mass is below the spring’s initial equilibrium position of x = 0. Note that if d were greater than h, X_min would be above x = 0, and the restoring force would act in the downward direction until the mass descended once more below x = 0.

According to Hooke’s Law, the restoring force decreases in magnitude as the spring is compressed. Consequently, the net force downward is greatest at X = X_min and the net force upward is greatest at X = X_max.

Energy

The mechanical energy of the vertically oscillating spring is:

KE = U⁹ + U^s

where U^g is gravitational potential energy and U^s is the spring’s (elastic) potential energy.

Note that the velocity of the block is zero at X = X_min and X = X_max , and maximized at the equilibrium position, x = –h. Consequently, the kinetic energy of the spring is zero for X = X_max and X = X_min and is greatest at x = –h. The gravitational potential energy of the system increases with the height of the mass. The elastic potential energy of the spring is greatest when the spring is maximally extended at X = X_max and decreases with the extension of the spring.

How This Knowledge Will Be Tested

Most of the questions on subject test Physics that deal with spring motion will ask qualitatively about the energy or velocity of a vertically oscillating spring. For instance, you may be shown a diagram capturing one moment in a spring’s trajectory and asked about the relative magnitudes of the gravitational and elastic potential energies and kinetic energy. Or you may be asked at what point in a spring’s trajectory the velocity is maximized. The answer, of course, is that it is maximized at the equilibrium position. It is far less likely that you will be asked a question that involves any sort of calculation.

Next to display next topic in the chapter.